Page 31 - Genetics_From_Genes_to_Genomes_6th_FULL_Part1

2.2 Genetic Analysis According to Mendel 23

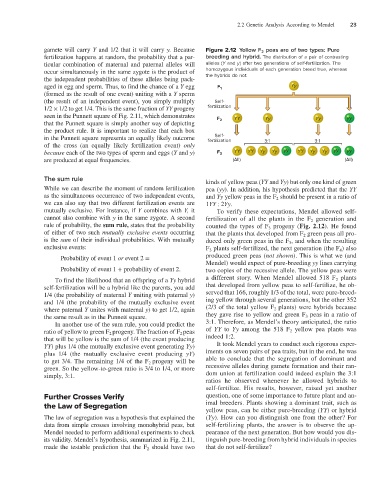
gamete will carry Y and 1/2 that it will carry y. Because Figure 2.12 Yellow F 2 peas are of two types: Pure
fertilization happens at random, the probability that a par- breeding and hybrid. The distribution of a pair of contrasting
ticular combination of maternal and paternal alleles will alleles (Y and y) after two generations of self-fertilization. The
occur simultaneously in the same zygote is the product of homozygous individuals of each generation breed true, whereas
the independent probabilities of these alleles being pack- the hybrids do not.
aged in egg and sperm. Thus, to find the chance of a Y egg F 1 Yy
(formed as the result of one event) uniting with a Y sperm
(the result of an independent event), you simply multiply Self-
1/2 × 1/2 to get 1/4. This is the same fraction of YY progeny fertilization
seen in the Punnett square of Fig. 2.11, which demonstrates F YY Yy Yy yy
that the Punnett square is simply another way of depicting 2
the product rule. It is important to realize that each box
Self-
in the Punnett square represents an equally likely outcome fertilization 3:1 3:1
of the cross (an equally likely fertilization event) only
because each of the two types of sperm and eggs (Y and y) F 3 YY YY Yy Yy yy YY Yy Yy yy yy
are produced at equal frequencies. (All) (All)

The sum rule kinds of yellow peas (YY and Yy) but only one kind of green
While we can describe the moment of random fertilization pea (yy). In addition, his hypothesis predicted that the YY
as the simultaneous occurrence of two independent events, and Yy yellow peas in the F 2 should be present in a ratio of
we can also say that two different fertilization events are 1YY : 2Yy.
mutually exclusive. For instance, if Y combines with Y, it To verify these expectations, Mendel allowed self-
cannot also combine with y in the same zygote. A second fertilization of all the plants in the F 2 generation and
rule of probability, the sum rule, states that the probability counted the types of F 3 progeny (Fig. 2.12). He found
of either of two such mutually exclusive events occurring that the plants that developed from F 2 green peas all pro-
is the sum of their individual probabilities. With mutually duced only green peas in the F 3 , and when the resulting
exclusive events: F 3 plants self- fertilized, the next generation (the F 4 ) also
Probability of event 1 or event 2 = produced green peas (not shown). This is what we (and
Mendel) would expect of pure-breeding yy lines carrying
Probability of event 1 + probability of event 2. two copies of the recessive allele. The yellow peas were
To find the likelihood that an offspring of a Yy hybrid a different story. When Mendel allowed 518 F 2 plants
self-fertilization will be a hybrid like the parents, you add that developed from yellow peas to self-fertilize, he ob-
1/4 (the probability of maternal Y uniting with paternal y) served that 166, roughly 1/3 of the total, were pure-breed-
and 1/4 (the probability of the mutually exclusive event ing yellow through several generations, but the other 352
where paternal Y unites with maternal y) to get 1/2, again (2/3 of the total yellow F 2 plants) were hybrids because
the same result as in the Punnett square. they gave rise to yellow and green F 3 peas in a ratio of
In another use of the sum rule, you could predict the 3:1. Therefore, as Mendel’s theory anticipated, the ratio
ratio of yellow to green F 2 progeny. The fraction of F 2 peas of YY to Yy among the 518 F 2 yellow pea plants was
that will be yellow is the sum of 1/4 (the event producing indeed 1:2.
YY) plus 1/4 (the mutually exclusive event generating Yy) It took Mendel years to conduct such rigorous exper-
plus 1/4 (the mutually exclusive event producing yY) iments on seven pairs of pea traits, but in the end, he was
to get 3/4. The remaining 1/4 of the F 2 progeny will be able to conclude that the segregation of dominant and
green. So the yellow-to-green ratio is 3/4 to 1/4, or more recessive alleles during gamete formation and their ran-
simply, 3:1. dom union at fertilization could indeed explain the 3:1
ratios he observed whenever he allowed hybrids to
self-fertilize. His results, however, raised yet another
Further Crosses Verify question, one of some importance to future plant and an-
the Law of Segregation imal breeders. Plants showing a dominant trait, such as
yellow peas, can be either pure-breeding (YY) or hybrid
The law of segregation was a hypothesis that explained the (Yy). How can you distinguish one from the other? For
data from simple crosses involving monohybrid peas, but self-fertilizing plants, the answer is to observe the ap-
Mendel needed to perform additional experiments to check pearance of the next generation. But how would you dis-
its validity. Mendel’s hypothesis, summarized in Fig. 2.11, tinguish pure-breeding from hybrid individuals in species
made the testable prediction that the F 2 should have two that do not self-fertilize?