Page 73 - Genetics_From_Genes_to_Genomes_6th_FULL_Part1

3.2 Extensions to Mendel for Two-Gene Inheritance 65

categories, for the sake of simplicity, we have looked at Although the possibilities for variation are manifold,
examples in which one allele of each gene in a pair showed none of the observed departures from Mendelian phenotypic
complete dominance over the other. But for any type of ratios contradicts Mendel’s genetic laws of segregation and
gene interaction, the alleles of one or both genes may ex- independent assortment. The alleles of each gene still seg-
hibit incomplete dominance or codominance, and these regate as he proposed. Interactions between the alleles of
possibilities increase the potential for phenotypic diversity. many genes simply make it harder to unravel the complex
For example, Fig. 3.21 shows how incomplete dominance relation of genotype to phenotype.
at both genes in a dihybrid cross results not in a collapse of
several genotypic classes into one but rather an expansion—
each of the nine genotypes in the dihybrid cross F 2 corre-
sponds to a different phenotype. Breeding Studies Help Geneticists
A simple biochemical explanation for the pheno- Determine Whether One or Two Genes
types in Fig. 3.21 is similar to that for incomplete domi- Determine a Trait
nance in Fig. 3.3b, where the amount of red pigment
produced was proportional to the amount of an enzyme. How do geneticists know whether a particular trait is
The difference here is that purple pigmentation requires caused by the alleles of one gene or by two genes interact-
the action of two enzymes, A and B, and one is more ef- ing in one of a number of possible ways? Breeding tests
ficient than the other, resulting in one gene (in this case, can usually resolve the issue. Phenotypic ratios diagnostic
the A gene) contributing more to the purple phenotype of a particular mode of inheritance (for instance, the 9:7
than the other gene. or 13:3 ratios indicating that two genes are interacting)
can provide the first clues and suggest hypotheses. Fur-
ther breeding studies can then show which hypothesis is
correct.
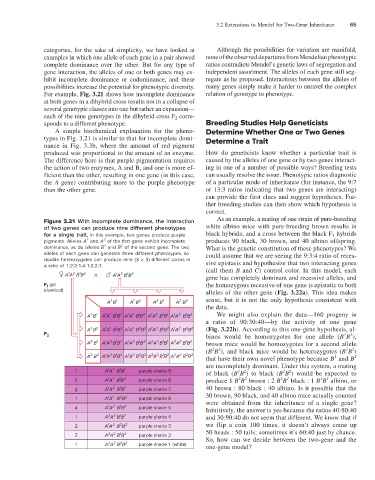
Figure 3.21 With incomplete dominance, the interaction As an example, a mating of one strain of pure-breeding
of two genes can produce nine different phenotypes white albino mice with pure-breeding brown results in
for a single trait. In this example, two genes produce purple black hybrids; and a cross between the black F 1 hybrids
2
1
pigments. Alleles A and A of the first gene exhibit incomplete produces 90 black, 30 brown, and 40 albino offspring.
1
2
dominance, as do alleles B and B of the second gene. The two What is the genetic constitution of these phenotypes? We
alleles of each gene can generate three different phenotypes, so could assume that we are seeing the 9:3:4 ratio of reces-
double heterozygotes can produce nine (3 × 3) different colors in sive epistasis and hypothesize that two interacting genes
a ratio of 1:2:2:1:4:1:2:2:1.
(call them B and C) control color. In this model, each
1 2
1 2
1 2
1 2
A A B B A A B B gene has completely dominant and recessive alleles, and
F (all the homozygous recessive of one gene is epistatic to both
1
identical) alleles of the other gene (Fig. 3.22a). This idea makes
1
2
2
1
A B 1 A B 2 A B 1 A B 2 sense, but it is not the only hypothesis consistent with
the data.
1 2
1 2
1 1
1 1
1 2
1 2
1 1
1
1 1
A B 1 A A B B A A B B A A B B A A B B We might also explain the data—160 progeny in
a ratio of 90:30:40—by the activity of one gene
1 2
1 1
2 2
1 2
1
1 1
2 2
1 2
1 2
A B 2 A A B B A A B B A A B B A A B B (Fig. 3.22b). According to this one-gene hypothesis, al-
F 2 binos would be homozygotes for one allele (B B ),
1 1
2 2
2 2
1 1
1 1
1 2
1 2
1 2
1 2
2
A B 1 A A B B A A B B A A B B A A B B brown mice would be homozygotes for a second allele
1 2
(B B ), and black mice would be heterozygotes (B B )
2 2
2 2
2 2
2 2
2
1 2
1 2
2 2
1 2
1 2
A B 2 A A B B A A B B A A B B A A B B that have their own novel phenotype because B and B
2
1
are incompletely dominant. Under this system, a mating
1 1
1 1
1 A A B B purple shade 9 of black (B B ) to black (B B ) would be expected to
1 2
1 2
1 2
2 2
1 1
1 1
1 1
2 A A B B purple shade 8 produce 1 B B brown : 2 B B black : 1 B B albino, or
1 1
1 2
2 A A B B purple shade 7 40 brown : 80 black : 40 albino. Is it possible that the
2 2
1 1
1 A A B B purple shade 6 30 brown, 90 black, and 40 albino mice actually counted
were obtained from the inheritance of a single gene?
1 2
1 2
4 A A B B purple shade 5 Intuitively, the answer is yes because the ratios 40:80:40
2 2
1 1
1 A A B B purple shade 4 and 30:90:40 do not seem that different. We know that if
1 2
2 2
2 A A B B purple shade 3 we flip a coin 100 times, it doesn’t always come up
2 2
1 2
2 A A B B purple shade 2 50 heads : 50 tails; sometimes it’s 60:40 just by chance.
So, how can we decide between the two-gene and the
2 2
2 2
1 A A B B purple shade 1 (white) one-gene model?